Struggling with calculus? You are not alone! Lots of IB students find it difficult but with the right breakdown, it’s easier than you think!
Why Does Calculus Matter in IB?
Calculus may be one of the trickiest concepts to master—but it’s also one of the most powerful. With the right understanding, you can apply it across a wide range of subjects and real-life problems.
It allows us to:
- Understand how things change (differentiation)
- Calculate areas and accumulated quantities (integration)
- Solve real-world problems in physics, economics, and medicine
Many students struggle because they focus on memorising formulas, rather than understanding concepts. In this guide, we’ll simplify calculus step by step.
What is Calculus, Really?
Before diving in, let’s clarify what calculus is all about.
Calculus is an area of mathematics that studies change and motion. Dissimilar to algebra which deals with static equations, calculus allows us to:
- Measure the rate at which things change (differentiation)
- Find the total amount of something accumulated over time (integration)
Real-World Applications of Calculus:
- Physics: Calculating speed, acceleration, and motion
- Biology: Measuring population growth
- Medicine: Calculating drug concentration in the bloodstream
Differentiation Explained Simply
Differentiation is the rate of change of a function at any given point. Simply, it tells us how fast something is changing at a certain point.
Differentiation can be written in two different ways: one way is as: d/dx f(x) and the other is f'(x), both of which represent the derivative of f(x).
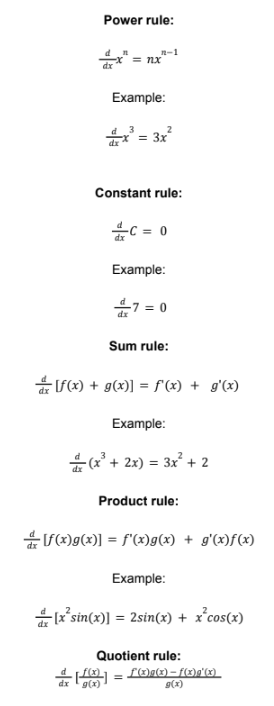
Basic Rules of Differentiation:
These are the most common rules that are used to differentiate a function:
Applications of Differentiation:
- Finding the slope of a curve at a point
- Identifying maximum and minimum values in optimisation problems
- Calculating rates of change in real world problems (e.g. population growth)
Integration Broken Down Simply
Integration is essentially the reverse of differentiation. It helps us calculate areas under curves and accumulated totals over time.
Basic Rules of Integration:
Definite vs Indefinite Integrals
- Indefinite Integrals don’t have limits; they give a general function + C
- Definite Integrals have limits and give an area or value, e.g.
Applications of Integration:
- Calculating area under a curve
- Solving physics problems (e.g. displacement from velocity)
Common IB Calculus Mistakes
❌ Forgetting the +C in indefinite integrals
❌ Not fully simplifying answers
❌ Confusing integration with differentiation
IB Calculus Study Tips
✅ Understand, don’t just memorise—know why the rules work
✅ Practise using IB past papers
✅ Use graphing calculators or apps like Desmos to visualise problems
Conclusion
Once you break it down into manageable steps, calculus becomes much less intimidating. Focus on understanding the why, practise regularly, and don’t hesitate to ask for help when needed. With time and strategy, you can master IB calculus and boost your confidence in Maths.
If you found these tips helpful, I’d personally love to support you as your tutor this year. You can view my profile here on Learnmate and contact me to discuss tutoring.
Alternatively, you can also engage other tutors on Learnmate to help you refine your IB Biology skills, boost your confidence, and provide guidance tailored to your specific needs. Connect with an expert IB Maths tutor on Learnmate and take your approach to the next level!